Geany 配置 Python
本文共 222 字,大约阅读时间需要 1 分钟。
我的电脑里有两个 Python,一个是平常的 Python,是在 Python 官网上下载的;另一个是在 MiniConda 上带来的。
平时用的是平常的 Python,但有一天想改成 miniconda 的 python,此时就要修改一下了:
首先: Build -> Set Build Conmmands
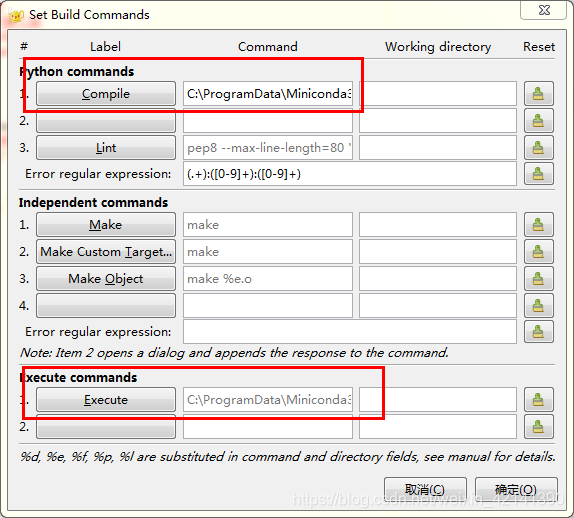
然后修改 Compile 和 Execute,将 -m 前和 “%f” 前 的 py 改成你想配置的 Python.exe 的路径:
转载地址:http://eqsg.baihongyu.com/
你可能感兴趣的文章
MySQL 树形结构 根据指定节点 获取其下属的所有子节点(包含路径上的枝干节点和叶子节点)...
查看>>
mysql 死锁 Deadlock found when trying to get lock; try restarting transaction
查看>>
mysql 死锁(先delete 后insert)日志分析
查看>>
MySQL 死锁了,怎么办?
查看>>
MySQL 深度分页性能急剧下降,该如何优化?
查看>>
MySQL 深度分页性能急剧下降,该如何优化?
查看>>
MySQL 添加列,修改列,删除列
查看>>
mysql 添加索引
查看>>
MySQL 添加索引,删除索引及其用法
查看>>
mysql 状态检查,备份,修复
查看>>
MySQL 用 limit 为什么会影响性能?
查看>>
MySQL 用 limit 为什么会影响性能?有什么优化方案?
查看>>
MySQL 用户权限管理:授权、撤销、密码更新和用户删除(图文解析)
查看>>
mysql 用户管理和权限设置
查看>>
MySQL 的 varchar 水真的太深了!
查看>>
mysql 的GROUP_CONCAT函数的使用(group_by 如何显示分组之前的数据)
查看>>
MySQL 的instr函数
查看>>
MySQL 的mysql_secure_installation安全脚本执行过程介绍
查看>>
MySQL 的Rename Table语句
查看>>
MySQL 的全局锁、表锁和行锁
查看>>